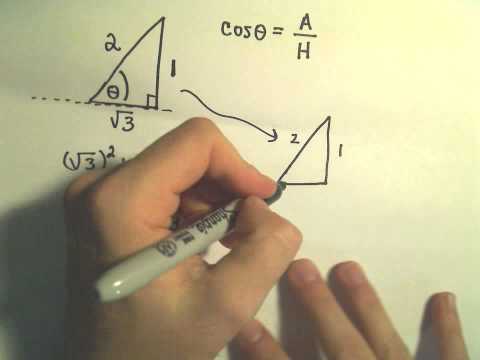
All right, in this video, we're going to do an example of finding the measure of an angle when we have some additional information. In this case, let's suppose we know that the cosine of theta is equal to the square root of 3 over 2. We want to find the measure of angle theta. Remember, the cosine of an angle is defined as the adjacent side over the hypotenuse. For our angle theta, the adjacent side can be labeled as the square root of 3, and the hypotenuse has a length of 2. Now we need to figure out the missing side, which we can find using the Pythagorean theorem. So, if we take the square root of 3 squared plus the missing side, which we'll call B squared, that equals 2 squared. Simplifying, we get 3 plus B squared equals 4. Subtracting 3 from both sides, we find that B squared equals 1. Taking the square root, we get both positive and negative 1, but since we're talking about a length, we choose the positive 1. Therefore, the missing side has a length of 1. Now, our goal is to find the measure of angle theta. To do this, let's get a little clever. We'll reflect this triangle over to the other side. Now we have an equilateral triangle, where all sides have the same length. Since the length of each side is 2, we know that all angles inside this equilateral triangle are the same. Since the total sum of the angles in a triangle is 180 degrees, each angle in this equilateral triangle must be 60 degrees. However, we are interested in angle theta, which was initially sitting in the upper region. This entire angle that traces out is 60 degrees. Therefore, angle theta is only half of that, which...
Award-winning PDF software





Video instructions and help with filling out and completing Which Form 2220 Determining