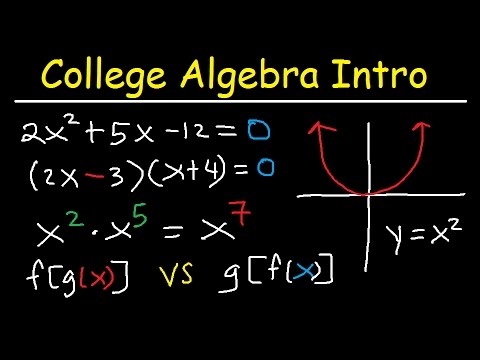
In this video, we're going to go over a basic introduction and overview of college algebra. So let's begin with some basics. What is x squared times x to the fifth power? When you multiply common bases, you need to add the exponents. x squared times x to the fifth power is x to the seventh power because 2 + 5 equals 7. X squared can be thought of as x times x. So when you multiply 2x variables together, you get x to the fifth power, which represents 5x variables combined. Now let's talk about division. What is x to the fifth power divided by x squared? When you divide, you need to subtract the exponents. 5 minus 2 equals 3. So x to the fifth power divided by x squared is equal to x cubed. Let's try another example. What is x to the fourth divided by x to the seventh? Subtracting the exponents, 4 minus 7 equals -3. Whenever you have a negative exponent, you can move the x variable from the top to the bottom and change the sign. So x to the fourth divided by x to the seventh is equal to 1 over x cubed. Now, what is x cubed raised to the fourth power? Whenever you raise an exponent to another exponent, you can multiply them. 3 times 4 equals 12. So the answer is x to the 12th power. When you raise something to the zero power, it's always equal to one. Now let's talk about simplifying expressions and combining like terms. If you were to see an expression like 5x + 3 + 7x - 4, how would you simplify it? The 5x and 7x are like terms, so you can add them together. 5 plus 7 is 12. So 5x...
Award-winning PDF software





Video instructions and help with filling out and completing Which Form 2220 Summary